Skillnad mellan versioner av "1.8 Talsystem med olika baser"

Från Mathonline
Hoppa till: navigering, sök
m
m
Rad 1: Rad 1:
 +
__NOTOC__
 
{| border="0" cellspacing="0" cellpadding="0" height="30" width="100%"
 
{| border="0" cellspacing="0" cellpadding="0" height="30" width="100%"
 
| style="border-bottom:1px solid #797979" width="5px" |  
 
| style="border-bottom:1px solid #797979" width="5px" |  
Rad 9: Rad 10:
  
  
−
__NOTOC__
+
<big>Om vårt talsystem med basen <math> \, 10 \, </math> se avsnittet [[1.1_Om_tal#Exempel_1|<b><span style="color:blue">Om tal, Exempel 1</span></b>]]<span style="color:black">:</span> <math> \; \boxed{ \; {\color{Red} {7\,142}} \; = \; {\color{Red} 7}\cdot 10\,^3\,+\,{\color{Red} 1}\cdot 10\,^2\,+\,{\color{Red} 4}\cdot 10\,^1\,+\,{\color{Red} 2}\cdot 10\,^0 \; } \; = \; ({\color{Red} {7\,142}})_{\text{tio}} </math> </big>
−
<big>Om vårt talsystem med basen <math> \, 10 \, </math> se avsnittet [[1.1_Om_tal#Exempel_1|<b><span style="color:blue">Om tal, Exempel 1</span></b>]]<span style="color:black">:</span> <math> \qquad \boxed{ \quad {\color{Red} {7\,142}} \; = \; {\color{Red} 7}\cdot 10\,^3\,+\,{\color{Red} 1}\cdot 10\,^2\,+\,{\color{Red} 4}\cdot 10\,^1\,+\,{\color{Red} 2}\cdot 10\,^0 \quad } </math> </big>
+
  
−
:::::::::::::::::::::::::::::<big><math> {\color{Red} 7} \cdot \;\, 8\,^3\,\,+\,{\color{Red} 1}\cdot \;\, 8\,^2\,+\,{\color{Red} 4}\cdot \;\, 8\,^1\,\,+\,{\color{Red} 2}\cdot \;\, 8\,^0 \quad = \; ({\color{Red} {7\,142}})_8 \; = \; 3\,682</math></big>
+
<big>Samma <span style="color:red">koefficienter</span> i talsystemet med basen <math> \, 8 \, </math> ger ett annat tal<span style="color:black">:</span> <math> \qquad\qquad\quad\;\, {\color{Red} 7} \cdot \;\, 8\,^3\,\,+\,{\color{Red} 1}\cdot \;\, 8\,^2\,+\,{\color{Red} 4}\cdot \;\, 8\,^1\,\,+\,{\color{Red} 2}\cdot \;\, 8\,^0 \;\;\; = \; ({\color{Red} {7\,142}})_{\text{åtta}} \; = \; (3\,682)_{\text{tio}}</math></big>
  
−
== <b><span style="color:#931136">Hur skriver man ett antal objekt med olika baser?</span></b> ==
+
== <b><span style="color:#931136">Omvandling från basen <math> \, 10 \, </math> till andra baser</span></b> ==
  
−
=== <b><span style="color:#931136">T.ex. 25 objekt med olika baser:</span></b> ===
+
=== <b><span style="color:#931136">Ex.<span style="color:black">:</span> <math> \;\; </math> Skriv <math> \, 25 \, </math> till andra baser.</span></b> ===
  
 
<div style="border:1px solid black;display:inline-table;margin-left: 50px;"> [[Image: Talsystem med olika baser_1_500.jpg]] </div>
 
<div style="border:1px solid black;display:inline-table;margin-left: 50px;"> [[Image: Talsystem med olika baser_1_500.jpg]] </div>
  
  
−
== <b><span style="color:#931136">Omvända problemet:</span></b> ==
+
== <b><span style="color:#931136">Omvandling från andra baser till basen <math> \, 10 \, </math>:</span></b> ==
  
−
=== <b><span style="color:#931136">T.ex.: Hur många objekt är <math> \, \bf{(34)_{sju}} \, </math>?</span></b> ===
+
=== <b><span style="color:#931136">Ex.<span style="color:black">:</span> <math> \;\; </math> Skriv <math> \, \bf{(34)_{sju}} \, </math> till basen <math> \, 10 \, </math>.</span></b> ===
  
 
<div style="border:1px solid black;display:inline-table;margin-left: 50px;"> [[Image: Talsystem med olika baser_2_500.jpg]] </div>
 
<div style="border:1px solid black;display:inline-table;margin-left: 50px;"> [[Image: Talsystem med olika baser_2_500.jpg]] </div>
Rad 40: Rad 40:
  
  
−
[[Matte:Copyrights|Copyright]] © 2011-2016 Math Online Sweden AB. All Rights Reserved.
+
[[Matte:Copyrights|Copyright]] © 2011-2017 Math Online Sweden AB. All Rights Reserved.

Versionen från 21 augusti 2017 kl. 20.15

       \( \pmb{\gets} \) Förra avsnitt          Genomgång          Övningar          Diagnosprov \( \pmb{\to} \)      


Om vårt talsystem med basen \( \, 10 \, \) se avsnittet Om tal, Exempel 1: \( \; \boxed{ \; {\color{Red} {7\,142}} \; = \; {\color{Red} 7}\cdot 10\,^3\,+\,{\color{Red} 1}\cdot 10\,^2\,+\,{\color{Red} 4}\cdot 10\,^1\,+\,{\color{Red} 2}\cdot 10\,^0 \; } \; = \; ({\color{Red} {7\,142}})_{\text{tio}} \)

Samma koefficienter i talsystemet med basen \( \, 8 \, \) ger ett annat tal: \( \qquad\qquad\quad\;\, {\color{Red} 7} \cdot \;\, 8\,^3\,\,+\,{\color{Red} 1}\cdot \;\, 8\,^2\,+\,{\color{Red} 4}\cdot \;\, 8\,^1\,\,+\,{\color{Red} 2}\cdot \;\, 8\,^0 \;\;\; = \; ({\color{Red} {7\,142}})_{\text{åtta}} \; = \; (3\,682)_{\text{tio}}\)

Omvandling från basen \( \, 10 \, \) till andra baser

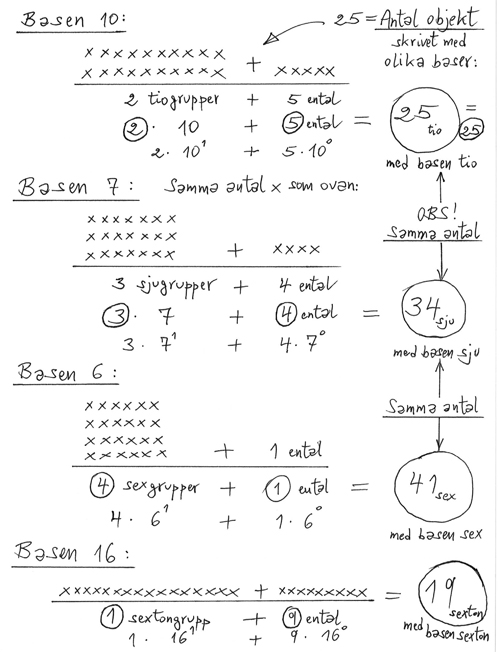
Ex.: \( \;\; \) Skriv \( \, 25 \, \) till andra baser.

Talsystem med olika baser 1 500.jpg


Omvandling från andra baser till basen \( \, 10 \, \):

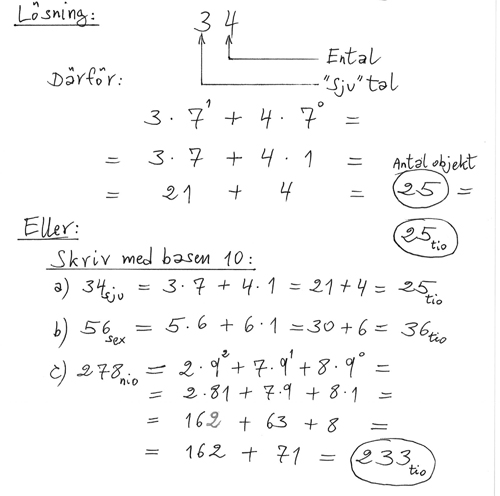
Ex.: \( \;\; \) Skriv \( \, \bf{(34)_{sju}} \, \) till basen \( \, 10 \, \).

Talsystem med olika baser 2 500.jpg


Omvandling mellan olika baser


Talsystem med olika baser 3 500.jpg





Copyright © 2011-2017 Math Online Sweden AB. All Rights Reserved.

Hämtad från "https://matte1b.mathonline.se/index.php?title=1.8_Talsystem_med_olika_baser&oldid=34241"