Skillnad mellan versioner av "1.8 Talsystem med olika baser"

Versionen från 22 september 2024 kl. 15.11

Genomgång

Det decimala talsystemet med basen \( \, 10 \, \) ger: \( \;\, \boxed{ \; {\color{Red} {7\,142}} \; = \; {\color{Red} 7}\cdot 10\,^3\,+\,{\color{Red} 1}\cdot 10\,^2\,+\,{\color{Red} 4}\cdot 10\,^1\,+\,{\color{Red} 2}\cdot 10\,^0 \; } \; = \; ({\color{Red} {7\,142}})_{\text{tio}} \)

Samma koefficienter med basen \( \, 8 \, \) ger ett annat tal: \( \qquad\quad\; {\color{Red} 7} \cdot \;\, 8\,^3\,\,+\,{\color{Red} 1}\cdot \;\, 8\,^2\,+\,{\color{Red} 4}\cdot \;\, 8\,^1\,\,+\,{\color{Red} 2}\cdot \;\, 8\,^0 \;\;\; = \; ({\color{Red} {7\,142}})_{\text{åtta}} \; = \; (3\,682)_{\text{tio}}\)

Resultat: Det decimala talet 3 682 är i det oktala talsystemet 7 142.

Omvandling från andra baser till basen \( \, 10 \, \)

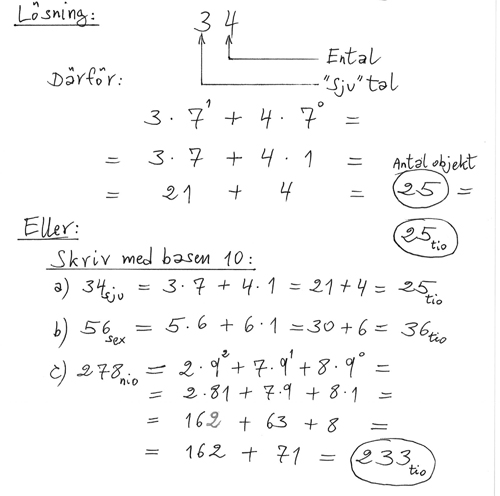
Uppgift: \( \, \) Skriv talet \( \, \bf{(34)_{sju}} \, \) från basen \( \, 7 \, \) till basen \( \, 10 \, \)

Omvandling från basen \( \, 10 \, \) till andra baser

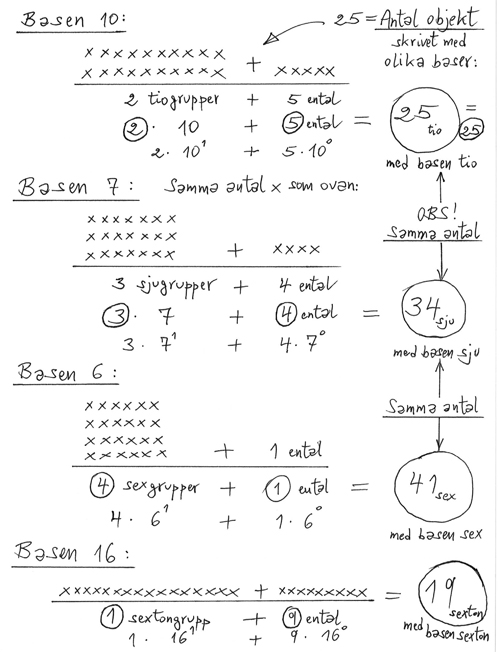
Exempel 1: \( \;\; \) Skriv \( \, 25 \, \) från basen \( \, 10 \, \) till andra baser, t.ex. \( \, 7 \, \), \( \, 6 \, \) och \( \, 16 \, \)

Exempel 2: \( \;\; \) Skriv \( \, 19 \, \) från basen \( \, 10 \, \) till basen \( \, 2 \, \)

Omvandling mellan olika baser

@@ Rad 11: / Rad 11: @@
-<big>Det [[1.1_Om_tal#Exempel_1|<b><span style="color:blue">decimala talsystemet</span></b>]] med basen <math> \, 10 \, </math> ger<span>:</span> <math> \;\, \boxed{ \; {\color{Red} {7\,142}} \; = \; {\color{Red} 7}\cdot 10\,^3\,+\,{\color{Red} 1}\cdot 10\,^2\,+\,{\color{Red} 4}\cdot 10\,^1\,+\,{\color{Red} 2}\cdot 10\,^0 \; } \; = \; ({\color{Red} {7\,142}})_{\text{tio}} </math> </big>
+<big>Det [[1.1_Om_tal#Exempel_1|<b><span style="color:blue">decimala talsystemet</span></b>]] med basen <math> \, 10 \, </math> ger<span>:</span> <math> \;\, \boxed{ \; {\color{Red} {7\,142}} \; = \; {\color{Red} 7}\cdot 10\,^3\,+\,{\color{Red} 1}\cdot 10\,^2\,+\,{\color{Red} 4}\cdot 10\,^1\,+\,{\color{Red} 2}\cdot 10\,^0 \; } \; = \; ({\color{Red} {7\,142}})_{\text{tio}} </math>
-<big>Samma <span style="color:red">koefficienter</span> med basen <math> \, 8 \, </math> ger ett annat tal<span style="color:black">:</span> <math> \qquad\quad\; {\color{Red} 7} \cdot \;\, 8\,^3\,\,+\,{\color{Red} 1}\cdot \;\, 8\,^2\,+\,{\color{Red} 4}\cdot \;\, 8\,^1\,\,+\,{\color{Red} 2}\cdot \;\, 8\,^0 \;\;\; = \; ({\color{Red} {7\,142}})_{\text{åtta}} \; = \; (3\,682)_{\text{tio}}</math></big>
+Samma <span style="color:red">koefficienter</span> med basen <math> \, 8 \, </math> ger ett annat tal<span style="color:black">:</span> <math> \qquad\quad\; {\color{Red} 7} \cdot \;\, 8\,^3\,\,+\,{\color{Red} 1}\cdot \;\, 8\,^2\,+\,{\color{Red} 4}\cdot \;\, 8\,^1\,\,+\,{\color{Red} 2}\cdot \;\, 8\,^0 \;\;\; = \; ({\color{Red} {7\,142}})_{\text{åtta}} \; = \; (3\,682)_{\text{tio}}</math>
+Resultat: Det decimala talet 3 682 är i det oktala talsystemet 7 142. </big>
 == <b><span style="color:#931136">Omvandling från andra baser till basen <math> \, 10 \, </math></span></b> ==